Explore 2.8 How Nature Produces Order in Stars

Learning Objectives

By the time you have completed the 2.8 Introduction & Exploration Activities, you should be able to:

• Understand and be able to identify each of the following: normal star, mini star, nebula(ae), globule, proplyd, nuclear fusion.
• Analyze the birth of stars in terms of governing processes and system states separated by transitions; specifically, how gravity and fusion produce the normal star state from a globule in a nebula.
• Describe how a change in one of the following properties of a normal star will affect the other properties: mass/gravity, fusion rate, true brightness, & temperature (all of which are proportional to each other); and lifetime (which is inversely proportional to the other properties).

---

Scientific Terms/Concepts

Terms: nebula(ae), globule, proplyd, nuclear fusion, radioactive isotope, stable isotope, half-life.

Define and give an example of each term:

Answer
Answer
Answer
Answer
Answer

Forming Normal Stars from Nebulae. The Gravity – Fusion Tug-o’-War!

The general pattern of star formation—based on millions of telescope observations—is shown in the image below: nebulae form proplyd that form normal stars (and accompanying planetary systems, which we’ll discuss later). What produces this ordered sequence?

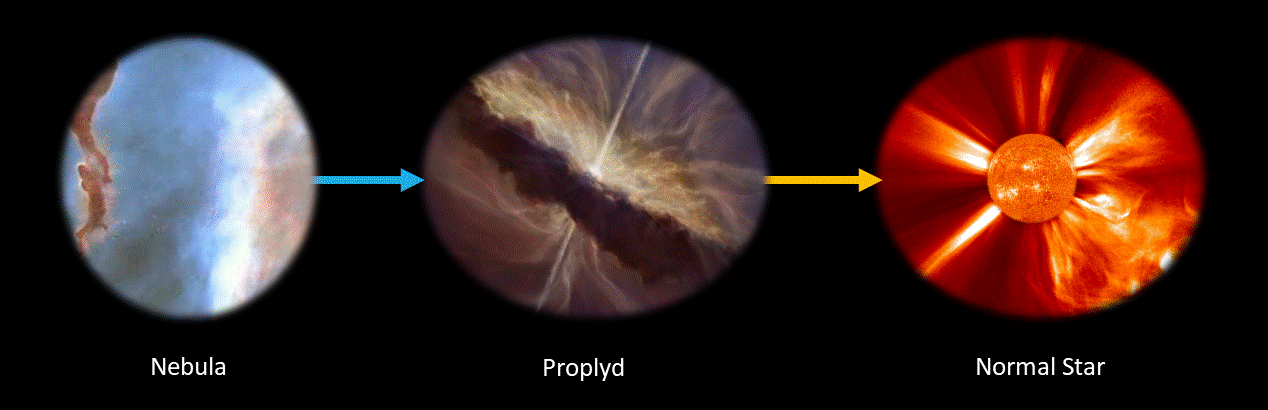
In the Introduction Activity, you learned that space contains clouds of gas and dust. These nebulae consist mostly of hydrogen (92%) and helium (8%) gas, with very small and relatively unabundant solid particles of dust. Gravitational interactions (caused by curved spacetime) in nebulae cause denser regions of gas (& dust) in a nebula to attract surrounding gas. And, as gas collects, the pull of gravity increases, which accelerates collapse. Under gravity alone, the gas and dust in a nebula would collapse to a point. However, the gas in nebulae is in motion and, as collapse proceeds, this motion increases.

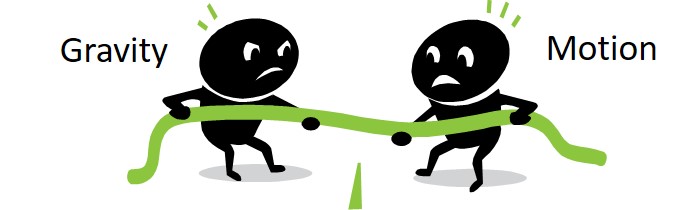
In case you don’t know, when you say, “That pot is hot!” what you mean is, “The atoms in that pot are vibrating—moving back and forth—energetically, with tremendous speed!” In other words, what we experience as ‘temperature’ results from the motion of atoms and molecules. As such, the following statements express the same idea: “The motion of gas, or gas pressure, in a collapsing nebula increases as collapse proceeds” and “The temperature of a collapsing nebula rises as collapse proceeds.” Thus, as gravity causes gas in a nebula to collapse, gas motion ≈ gas pressure ≈ temperature grows and opposes collapse. This is a very important idea, so we’ll say it again: increasing gas motion opposes—provides a negative feedback to—gravitational collapse. Said another way, there is ‘opposition in all things’—including in star formation. These opposing governing processes—which play such important roles in star formation, development, and demise—are illustrated in the cartoon below.

How does gravitational collapse inside nebulae begin? It can begin on its own, but more commonly gravitational collapse receives a boost from processes operating in galaxies. These processes, which we’ll discuss later in this unit, compress gas in nebulae, increasing its density. If compression-induced increases in density are sufficient, gravitational effects become strong enough to overcome motion and cause the cloud to collapse. Of course, cool nebulae are easier to compress than hot nebulae, because gas motion in hot nebula more strongly resists compression.

To help you build your intuition about the relationship between gravity and motion, consider the analogy illustrated in the cartoon above: Imagine a tug of war, with gravity as the team that pulls in one direction and motion as the team that pulls in the opposite direction. In a tug of war competition, each team tries to move the rope (and the other team!) across the center line (which is sometimes a mud puddle!).

These competitions can be dynamic, with one team succeeding in moving all competitors in their direction for a time, only to have the other team overcome them and move everyone in the opposite direction. And these periods of imbalance can sometimes be separated by long periods of stasis, where the efforts of opposing teams are equally balanced. These static periods can seem deceptively inactive. Despite the lack of movement, both teams continue working strenuously against each other. We invite you to pause for a moment and contemplate the analogy by thoughtfully exploring answers to these questions:

• What happens to the nebula when the gravity team dominates?
• What happens to the nebula when the motion team dominates?
• During periods of gravitational domination, what changes to nebula strengthen the efforts of the motion team?
• During periods of motion dominance, what changes to the nebula strengthen the efforts of the gravity team?
• If there were an instantaneous change in the strength of one team (e.g., the addition of another team member), how would the opposing team respond? Would their loss be ensured or could the initially-overcome team derive strength from the very change that originally overpowered them?

Thoughts about Learning. If you kind of skimmed through these questions, you’ll likely have robbed yourself of the opportunity for these important ideas to take root inside you. If you gave up because you found it frustrating not to know the answer, remember that learning is work and avoiding the work will weaken you. Also, recall that striving for an answer is, at least initially, far more important to learning that getting the right answer. Obviously, the choice is yours, but if you choose to deal shallowly with this material here, you’ll likely have lost the opportunity to exercise your agency—for, come test time, your need to perform will ‘act on you’ and force you into the distasteful memorize and purge mode of test prep. Have you ever wondered why we all hate learning in that mode so much? It’s because no one enjoys being acted upon; our agency is far too important to each of us. Learning is a deeply joyful and satisfying experience, but only when you’re in the driver’s seat. Choosing to be a passenger—viewing learning as a series of short trips in which the instructor drives you from one idea to the next—will never satisfy you; in fact, it will infuriate you. Whether you choose to be an active learner in this course or not, allow us to bless you with this gift: if you ever find yourself annoyed by learning, take a moment to assess your choices. In these situations, you will often discover that you’re irritated because you’ve placed someone else in the driver’s seat and you don’t like how they’re driving or the route they’ve chosen. Here’s the gift: the very act of recognizing the reason why your annoyed will provide you with the opportunity to recover your agency and begin again to enjoy the journey. Happy learning!

---

Gravity & Fusion during Star Formation

Within a collapsing nebula, the gravity team dominates the motion team, because the increasing density produced by collapse continuously strengthens the gravity. Said another way: During collapse, increasing density produces increased gravity, which generates increased density… in a positive feedback loop that continuously shrinks the collapsing portion of the nebula. Incidentally, astronomers refer to the collapsing portions of a nebula as globules. We won’t require that you memorize this term, but we’ll use it—so you ought to be at least familiar with it. For our purposes, more important than the term is the idea that nebulae don’t collapse to form a single star. They collapse to form numerous (often thousands) individual stars, and each solar system forms from a single globule.

During the extended period of gravitational dominance that drives globule collapse, ever increasing density produces ever higher gas motion ≈ gas pressure ≈ temperature. This causes atoms to collide with increasing energy, and these collisions increasingly resist gravitational collapse. When rising temperatures at the dense center of the globule reach 10 million degrees and rising gas pressures reach one billion times Earth’s atmospheric pressure, atomic collisions become so crushingly intense that proton collisions create new, larger nuclei. The process of joining lighter nuclei to form a heavier nucleus is called nuclear fusion. In the cores of normal stars, abundant (~92%) hydrogen nuclei—mostly individual protons—combine to form helium nuclei. In the process of producing helium nuclei from hydrogen nuclei, a small amount of matter (m, mass) becomes a tremendous amount of energy (E). The conversion is expressed in Einstein’s famous equation, E=mc², where c is the speed of light. The diagram below illustrates the tug of war between gravity and fusion energy ≈ gas pressure ≈ temperature.

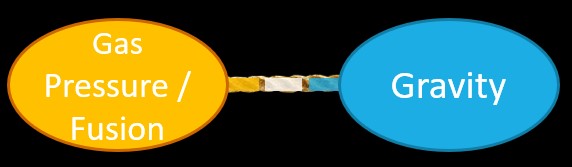
The energy released by nuclear fusion increases temperature and gas motion/pressure, which overcomes the inward pull of gravity and causes the star to expand. Expansion cools the star and decreases gas pressure, which eventually halts fusion. Without the additional energy provided by fusion, collapse begins again. In this process, the inward pull of gravity and the outward push produced by fusion energy form a negative feedback cycle causing ever-dampening sequences of collapse, initiation of fusion, expansion, cessation of fusion, collapse, ...—until the competing forces of gravity and gas pressure balance and a new star is born! Of course, other factors such as the in-falling of additional matter can provide additional instability for a time, but the negative feedbacks between gravity and fusion energy ensures eventual stability. That was important! Did you catch it? The negative feedback cycle of gravity and fusion energy ensure that, eventually, a stable normal star will emerge from collapsing globule and the yo-yo-like instabilities associated with star birth. Said another way, these processes (gravity and fusion energy) govern the production of an ordered state (a normal star) from a disordered state (a globule inside a nebula).

In addition to the balance of gravity and fusion energy, the energy production in the core of a star core must balance the energy radiated from the surface of the star. Without this equilibrium, the star’s interior would heat up—causing the core to expand—or the interior would cool down—causing the core to shrink. Thus, the star’s surface temperature and diameter must adjust so that it radiates the same amount of energy that fusion produces in the core. As a result, both the temperature and diameter of a normal star are determined by the star’s mass. As such, stars with more mass are hotter, more luminous, and have larger diameters than stars with less mass. Can you see how relationships between the properties of stars (such as mass, temperature, luminosity, and diameter) allow pushes to produce responses?

This balanced state—in which the outward push of energy from fusion of hydrogen in the core of the star is balanced by the inward pull of gravity—is known as a normal star. Our Sun is one such star. In essence, normal stars are huge, continuous nuclear explosions that are prevented from blowing apart by the balanced, counteracting tug of gravity. Relative to the lifetimes of normal stars, which extend from a few hundred thousand to trillions of years, the transition from the disordered state of nebulae to the ordered state of normal stars is relatively short. On average, formation—which begins with globule collapse and ends stable H fusion—occupies ~0.1 percent of the lifetime of a normal star.

As mentioned in the Introduction Activity, as a normal star is forming in the central dense region of a collapsing cloud, the spin of the globule and the energy loss (by radiation) of infalling matter produce the protoplanetary disk. Later in this unit, we’ll address the processes that produce planets inside the proplyd. To help you visualize the development of a nebula into several growing stars, watch this computer simulation (1.5 min).

Habitable worlds like Earth require a relatively constant, steady, long-lived source of energy. Without it, Earth’s dynamic systems would be unable to sustain life. Normal stars provide this long-lived, stable source of energy. Below, we provide you with a guided opportunity to deepen and reinforce your understanding of the important relationships between the characteristics and governing processes of stars that we discussed above.

Exploring General Relationships

Answer the questions below.

Answer

Answer

Answer

Answer

Answer

Answer

Comparing Spica & The Sun

Spica and the Sun are both normal stars. Spica is ~9.5 times more massive than the Sun. Answer the questions below about these two stars.

Answer

Answer

Answer

Comparing Barnard’s Star & The Sun

The Sun and Barnard’s star are both normal stars. The Sun is a yellow normal star. Barnard’s star is a red dwarf—a small, red, normal star. Answer the questions below about these two stars.

Answer

Answer

Answer

Summary. Relationships between Stellar Properties

The mass of stars is a fundamentally important property because mass determines most other characteristics of a star, including the strength of the gravitational pull, fusion rate, luminosity, temperature, diameter, and lifetime. Massive stars have stronger gravitational pulls (curve spacetime more); higher fusion rates, luminosities, and diameters; and shorter lifetimes. To many who are unfamiliar with stars, the inverse relationship between mass and lifetime appears counterintuitive. Their intuition leads them to believe that because massive stars have more fuel, they should live longer ‘lives’. Although it is true that massive stars have more fuel, it is also true that they have much higher fusion rates. In other words, massive stars consume fuel much faster than less massive stars. Thus, the least massive stars ‘live’ the longest and the most massive stars have the shortest lifetimes.

To help you understand the inverse relationship of stellar mass and lifetime, consider this analogy: Compare an enormous mine truck to a scooter. The mine truck has a 200 gallon tank (much more fuel) but burns fuel at the rate of 5 gallons per mile (seriously, its fuel efficiency is measured in gallons per mile!), whereas the scooter has a 1 gallon tank (much less fuel) but burns fuel at the rate of 100 miles per gallon. Which vehicle will run out of fuel first? The mine truck! Despite having 200 times more fuel, the mine truck burns its fuel 500 times faster than the scooter. Make sense?

---

---